Space & Physics
Why does superconductivity matter?

Superconductivity was discovered by H. Kamerlingh Onnes on April 8, 1911, who was studying the resistance of solid Mercury (Hg) at cryogenic temperatures. Liquid helium was recently discovered at that time. At T = 4.2K, the resistance of Hg disappeared abruptly. This marked a transition to a new phase that was never seen before. The state is resistanceless, strongly diamagnetic, and denotes a new state of matter. K. Onnes sent two reports to KNAW (the local journal of the Netherlands), where he preferred calling the zero-resistance state ‘superconductivity’’.
There was another discovery that went unnoticed in the same experiment, which was the transition of superfluid Helium (He) at 2.2K, the so-called λ transition, below which He becomes a superfluid. However, we shall skip that discussion for now. A couple of years later, superconductivity was found in lead (Pb) at 7K. Much later, in 1941, Niobium Nitride was found to superconduct below 16 K. The burning question in those days was: what would the conductivity or resistivity of metals be at a very low temperature?
The reason behind such a question is Lord Kelvin’s suggestion that for metals, initially the resistivity decreases with falling temperature and finally climbs to infinity at zero Kelvin because electrons’ mobility becomes zero at 0 K, yielding zero conductivity and hence infinite resistivity. Kamerlingh Onnes and his assistant Jacob Clay studied the resistance of gold (Au) and platinum (Pt) down to T = 14K. There was a linear decrease in resistance until 14 K; however, lower temperatures cannot be accessed owing to the unavailability of liquid He, which eventually happened in 1908.
In fact, the experiment with Au and Pt was repeated after 1908. For Pt, the resistivity became constant after 4.2K, while Au is found to superconduct at very low temperatures. Thus, Lord Kelvin’s notion about infinite resistivity at very low temperatures was incorrect. Onnes had found that at 3 K (below the transition), the normalised resistance is about 10−7. Above 4.2 K, the resistivity starts appearing again. The transition is too sharp and falls abruptly to zero within a temperature window of 10−4 K.
All superconductors are normal metals above the transition temperature. If we ask in the periodic table where most of the superconductors are located, the answer throws some surprises. The good metals are rarely superconducting
Perfect conductors, superconductors, and magnets
All superconductors are normal metals above the transition temperature. If we ask in the periodic table where most of the superconductors are located, the answer throws some surprises. The good metals are rarely superconducting. The examples are Ag, Au, Cu, Cs, etc., which have transition temperatures of the order of ∼ 0.1K, while the bad metals, such as niobium alloys, copper oxides, and 1 MgB2, have relatively larger transition temperatures. Thus, bad metals are, in general, good superconductors. An important quantity in this regard is the mean free path of the electrons. The mean free path is of the order of a few A0 for metals (above Tc), while for good metals (or the bad superconductors), it is usually a few hundred of A0. Whereas for the bad metals (good superconductors), it is still small as the electrons are strongly coupled to phonons. The orbital overlap is large in a superconductor. In good metals, the orbital overlap is small, and often they become good magnets. In the periodic table, transition elements such as the 3D series elements, namely Al, Bi, Cd, Ga, etc., become good superconductors, while Cr, Mn, and Fe are bad superconductors and in fact form good magnets. For all of them, that is, whether they are superconductors or magnets, there is a large density of states at the Fermi level. So, a lot of electronic states are necessary for the electrons in these systems to be able to condense into a superconducting state (or even a magnetic state). The nature of the electronic wave function determines whether they develop superconducting order or magnetic order. For example, electronic wavefunctions have a large spatial extent for superconductors, while they are short-range for magnets.
Meissner effect
The near-complete expulsion of the magnetic field from a superconducting specimen is called the Meissner effect. In the presence of a magnetic field, the current loops at the periphery will be generated so as to block the entry of the external field inside the specimen. If a magnetic field is allowed within a superconductor, then, by Ampere’s law, there will be normal current within the sample. However, there is no normal current inside the specimen. Thus, there can’t be any magnetic field. For this reason, superconductors are known as perfect diamagnets with very large diamagnetic susceptibility. Even the best-known diamagnets (which are non-superconductors) have magnetic susceptibilities of the order of 10−5. Thus, the diamagnetic property can be considered a distinct property of superconductors compared to zero electrical resistance.
The near-complete expulsion of the magnetic field from a superconducting specimen is called the Meissner effect
A typical experiment demonstrating the Meissner effect can be thought of as follows: Take a superconducting sample (T < Tc), sprinkle iron filings around the sample, and switch on the magnetic field. The iron filings are going to line up in concentric circles around the specimen. This implies the expulsion of the flux lines outside the sample, which makes the filings line up.
Distinction between perfect conductors and superconductors
The distinction between a perfect conductor and a superconductor is brought about by magnetic field-cooled (FC) and zero-field-cooled (ZFc) cases, as shown below in Fig. 1.
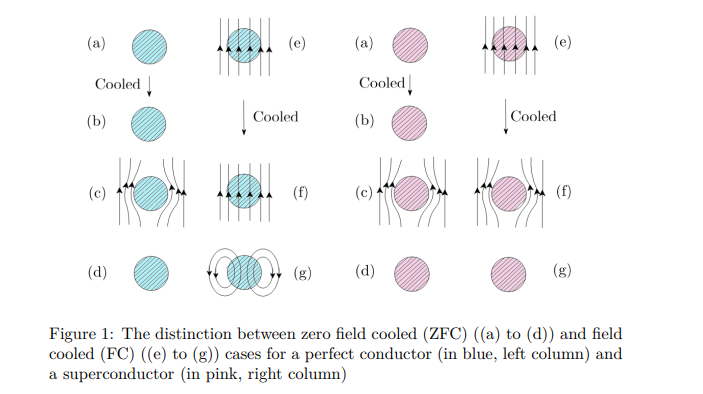
In the absence of an external magnetic field, temperature is lowered for both the metal and the superconductor in their metallic states from T > Tc to T < Tc (see left panel for both in Fig. 1). Hence, a magnetic field is applied, which eventually gets expelled owing to the Meissner effect. The field has finally been withdrawn. However, if cooling is done in the presence of an external field, after the field is withdrawn, the flux lines get trapped for a perfect conductor; however, the superconductor is left with no memory of an applied field, a situation similar to what happens in the zero-field cooling case. So, superconductors have no memory, while perfect conductors have memory.
Microscopic considerations: BCS theory
The first microscopic theory of superconductivity was proposed by Berdeen, Cooper, and Schrieffer (BCS) in 1957, which earned them a Nobel Prize in 1972. The underlying assumption was that an attractive interaction between the electrons is possible, which is mediated via phonons. Thus, electrons form bound pairs under certain conditions, such as (i) two electrons in the vicinity of the filled Fermi Sea within an energy range ¯hωD (set by the phonons or lattice). (ii) The presence of phonons or the underlying lattice is confirmed by the isotope effect experiment, which confirms that the transition temperature is proportional to the mass of ions. Since the Debye frequency depends on the ionic mass, it implies that the lattice must be involved. 3 A small calculation yields that an attractive interaction is possible in a narrow range of energy. This attractive interaction causes the system to be unstable, and a long-range order develops via symmetry breaking. In a book by one of the discoverers, namely, Schrieffer, he described an analogy between a dancing floor comprising couples, dancing one with any other couple, and being completely oblivious to any other couple present in the room. The couples, while dancing, drift from one end of the room to another but do not collide with each other. This implies less dissipation in the transport of a superconductor. The BCS theory explained most of the features of the superconductors known at that time, such as (i) the discontinuity of the specific heat at the transition temperature, Tc. (ii) Involvement of the lattice via the isotope effect. (iii) Estimation of Tc and the energy gap. The value of Tc and the gap are confirmed by tunnelling experiments across metal-superconductor (M-S) or metal-insulator-superconductor (MIS) types of junctions. Giaever was awarded the Nobel Prize in 1973 for his work on these experiments. (iv) The Meissner effect can be explained within a linear response regime. (v) Temperature dependence of the energy gap, confirming gradual vanishing, which confirms a second-order phase transition. Most of the features of conventional superconductors can be explained using BCS theory. Another salient feature of the theory is that it is non-perturbative. There is no small parameter in the problem. The calculations were done with a variational theory where the energy is minimised with respect to some free parameters of the variational wavefunction, known as the BCS wavefunction.
Unconventional Superconductors: High-Tc Cuprates
This is a class of superconductors where the two-dimensional copper oxide planes play the main role, and superconductivity occurs in these planes. Doping these planes with mobile carriers makes the system unstable towards superconducting correlations. At zero doping, the system is an antiferromagnetic insulator (see Fig. 2). With about 15% to 20% doping with foreign elements, such as strontium (Sr), etc. (for example, in La2−xSrxCuO4), the system turns superconductivity. There are two things that are surprising in this regard. (i) The proximity of the insulating state to the superconducting state; (ii) For the system initially in the superconducting state, as the temperature is raised, instead of going into a metallic state, it shows several unfamiliar features that are very unlike the known Fermi liquid characteristics. It is called a strange metal.
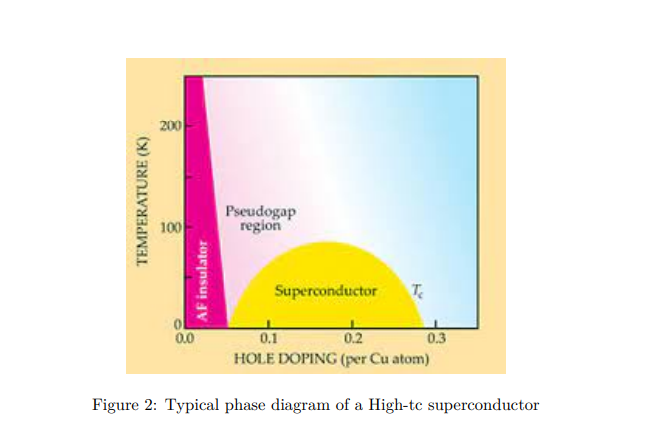
In fact, there are some signatures of pre-formed pairs in the ‘so-called’ metallic state, known as the pseudo gap phase. Since the starting point from which one should build a theory is missing, a complete understanding of the mechanism leading to the phenomenon cannot be understood. It remained a theoretical riddle.
Space & Physics
Nobel Prize in Physics: Clarke, Devoret, and Martinis Honoured for Pioneering Quantum Discoveries
The 2025 Nobel Prize in Physics honours John Clarke, Michel H. Devoret, and John M. Martinis for revealing how entire electrical circuits can display quantum behaviour — a discovery that paved the way for modern quantum computing.

The 2025 Nobel Prize in Physics has been awarded to John Clarke, Michel H. Devoret, and John M. Martinis for their landmark discovery of macroscopic quantum mechanical tunnelling and energy quantisation in an electric circuit, an innovation that laid the foundation for today’s quantum computing revolution.
Announcing the prize, Olle Eriksson, Chair of the Nobel Committee for Physics, said, “It is wonderful to be able to celebrate the way that century-old quantum mechanics continually offers new surprises. It is also enormously useful, as quantum mechanics is the foundation of all digital technology.”
The Committee described their discovery as a “turning point in understanding how quantum mechanics manifests at the macroscopic scale,” bridging the gap between classical electronics and quantum physics.
John Clarke: The SQUID Pioneer
British-born John Clarke, Professor Emeritus at the University of California, Berkeley, is celebrated for his pioneering work on Superconducting Quantum Interference Devices (SQUIDs) — ultra-sensitive detectors of magnetic flux. His career has been marked by contributions that span superconductivity, quantum amplifiers, and precision measurements.
Clarke’s experiments in the early 1980s provided the first clear evidence of quantum behaviour in electrical circuits — showing that entire electrical systems, not just atoms or photons, can obey the strange laws of quantum mechanics.
A Fellow of the Royal Society, Clarke has been honoured with numerous awards including the Comstock Prize (1999) and the Hughes Medal (2004).
Michel H. Devoret: Architect of Quantum Circuits
French physicist Michel H. Devoret, now the Frederick W. Beinecke Professor Emeritus of Applied Physics at Yale University, has been one of the intellectual architects of quantronics — the study of quantum phenomena in electrical circuits.
After earning his PhD at the University of Paris-Sud and completing a postdoctoral fellowship under Clarke at Berkeley, Devoret helped establish the field of circuit quantum electrodynamics (cQED), which underpins the design of modern superconducting qubits.
His group’s innovations — from the single-electron pump to the fluxonium qubit — have set performance benchmarks in quantum coherence and control. Devoret is also a recipient of the Fritz London Memorial Prize (2014) and the John Stewart Bell Prize, and is a member of the French Academy of Sciences.
John M. Martinis: Building the Quantum Processor
American physicist John M. Martinis, who completed his PhD at UC Berkeley under Clarke’s supervision, translated these quantum principles into the hardware era. His experiments demonstrated energy level quantisation in Josephson junctions, one of the key results now honoured by the Nobel Committee.
Martinis later led Google’s Quantum AI lab, where his team in 2019 achieved the world’s first demonstration of quantum supremacy — showing a superconducting processor outperforming the fastest classical supercomputer on a specific task.
A former professor at UC Santa Barbara, Martinis continues to be a leading voice in quantum computing research and technology development.
A Legacy of Quantum Insight
Together, the trio’s discovery, once seen as a niche curiosity in superconducting circuits, has become the cornerstone of the global quantum revolution. Their experiments proved that macroscopic electrical systems can display quantised energy states and tunnel between them, much like subatomic particles.
Their work, as the Nobel citation puts it, “opened a new window into the quantum behaviour of engineered systems, enabling technologies that are redefining computation, communication, and sensing.”
Space & Physics
The Tiny Grip That Could Reshape Medicine: India’s Dual-Trap Optical Tweezer
Indian scientists build new optical tweezer module—set to transform single-molecule research and medical Innovation
In an inventive leap that could open up new frontiers in neuroscience, drug development, and medical research, scientists in India have designed their own version of a precision laboratory tool known as the dual-trap optical tweezers system. By creating a homegrown solution to manipulate and measure forces on single molecules, the team brings world-class technology within reach of Indian researchers—potentially igniting a wave of scientific discoveries.
Optical tweezers, a Nobel Prize-winning invention from 2018, use focused beams of light to grab and move microscopic objects with extraordinary accuracy. The technique has become indispensable for measuring tiny forces and exploring the mechanics of DNA, proteins, living cells, and engineered nanomaterials. Yet, decades after their invention, conventional optical tweezers systems sometimes fall short for today’s most challenging experiments.
Researchers at the Raman Research Institute (RRI), an autonomous institute backed by India’s Department of Science and Technology in Bengaluru, have now introduced a smart upgrade that addresses long-standing pitfalls of dual-trap tweezers. Traditional setups rely on measuring the light that passes through particles trapped in two separate beams—a method prone to signal “cross-talk.” This makes simultaneous, independent measurement difficult, diminishing both accuracy and versatility.
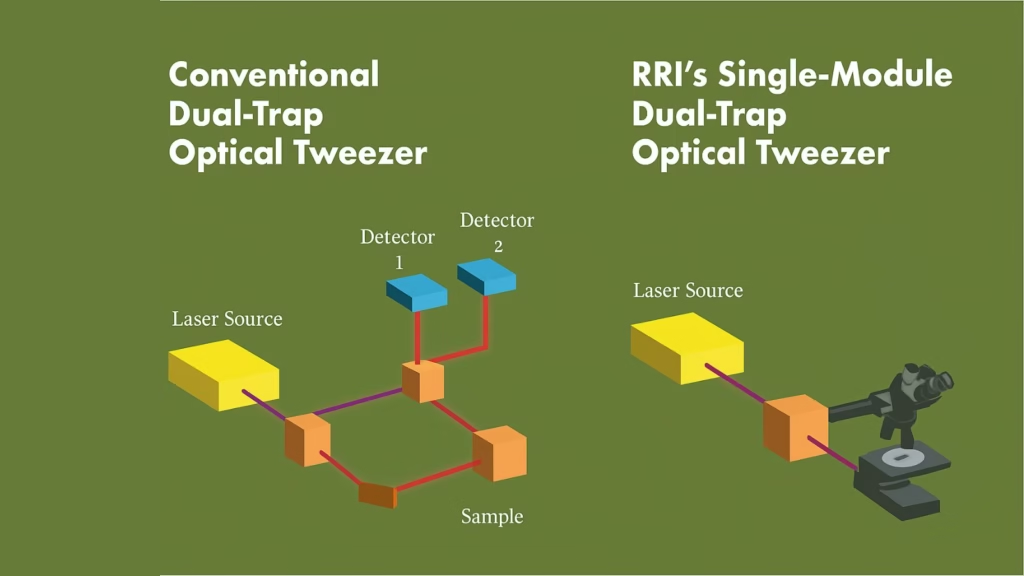
The new system pioneers a confocal detection scheme. In a media statement, Md Arsalan Ashraf, a doctoral scholar at RRI, explained, “The unique optical trapping scheme utilizes laser light scattered back by the sample for detecting trapped particle position. This technique pushes past some of the long-standing constraints of dual-trap configurations and removes signal interference. The single-module design integrates effortlessly with standard microscopy frameworks,” he said.
The refinement doesn’t end there. The system ensures that detectors tracking tiny particles remain perfectly aligned, even when the optical traps themselves move. The result: two stable, reliable measurement channels, zero interference, and no need for complicated re-adjustment mid-experiment—a frequent headache with older systems.
Traditional dual-trap designs have required costly and complex add-ons, sometimes even hijacking the features of laboratory microscopes and making additional techniques, such as phase contrast or fluorescence imaging, hard to use. “This new single-module trapping and detection design makes high-precision force measurement studies of single molecules, probing of soft materials including biological samples, and micromanipulation of biological samples like cells much more convenient and cost-effective,” said Pramod A Pullarkat, lead principal investigator at RRI, in a statement.
By removing cross-talk and offering robust stability—whether traps are close together, displaced, or the environment changes—the RRI team’s approach is not only easier to use but far more adaptable. Its plug-and-play module fits onto standard microscopes without overhauling their basic structure.
From the intellectual property point of view, this design may be a game-changer. By cracking the persistent problem of signal interference with minimalist engineering, the new setup enhances measurement precision and reliability—essential advantages for researchers performing delicate biophysical experiments on everything from molecular motors to living cells.
With the essential building blocks in place, the RRI team is now exploring commercial avenues to produce and distribute their single-module, dual-trap optical tweezer system as an affordable add-on for existing microscopes. The innovation stands to put advanced single-molecule force spectroscopy, long limited to wealthier labs abroad, into the hands of scientists across India—and perhaps spark breakthroughs across the biomedical sciences.
Space & Physics
New Magnetic Transistor Breakthrough May Revolutionize Electronics
A team of MIT physicists has created a magnetic transistor that could make future electronics smaller, faster, and more energy-efficient. By swapping silicon for a new magnetic semiconductor, they’ve opened the door to game-changing advancements in computing.
For decades, silicon has been the undisputed workhorse in transistors—the microscopic switches responsible for processing information in every phone, computer, and high-tech device. But silicon’s physical limits have long frustrated scientists seeking ever-smaller, more efficient electronics.
Now, MIT researchers have unveiled a major advance: they’ve replaced silicon with a magnetic semiconductor, introducing magnetism into transistors in a way that promises tighter, smarter, and more energy-saving circuits. This new ingredient, chromium sulfur bromide, makes it possible to control electricity flow with far greater efficiency and could even allow each transistor to “remember” information, simplifying circuit design for future chips.
“This lack of contamination enables their device to outperform existing magnetic transistors. Most others can only create a weak magnetic effect, changing the flow of current by a few percent or less. Their new transistor can switch or amplify the electric current by a factor of 10,” the MIT team said in a media statement. Their work, detailed in Physical Review Letters, outlines how this material’s stability and clean switching between magnetic states unlocks a new degree of control.
Chung-Tao Chou, MIT graduate student and co-lead author, explains in a media statement, “People have known about magnets for thousands of years, but there are very limited ways to incorporate magnetism into electronics. We have shown a new way to efficiently utilize magnetism that opens up a lot of possibilities for future applications and research.”
The device’s game-changing aspect is its ability to combine the roles of memory cell and transistor, allowing electronics to read and store information faster and more reliably. “Now, not only are transistors turning on and off, they are also remembering information. And because we can switch the transistor with greater magnitude, the signal is much stronger so we can read out the information faster, and in a much more reliable way,” said Luqiao Liu, MIT associate professor, in a media statement.
Moving forward, the team is looking to scale up their clean manufacturing process, hoping to create arrays of these magnetic transistors for broader commercial and scientific use. If successful, the innovation could usher in a new era of spintronic devices, where magnetism becomes as central to electronics as silicon is today.
-

 Space & Physics5 months ago
Space & Physics5 months agoIs Time Travel Possible? Exploring the Science Behind the Concept
-

 Earth6 months ago
Earth6 months ago122 Forests, 3.2 Million Trees: How One Man Built the World’s Largest Miyawaki Forest
-

 Space & Physics6 months ago
Space & Physics6 months agoDid JWST detect “signs of life” in an alien planet?
-

 Know The Scientist5 months ago
Know The Scientist5 months agoNarlikar – the rare Indian scientist who penned short stories
-

 Society4 months ago
Society4 months agoShukla is now India’s first astronaut in decades to visit outer space
-

 Society4 months ago
Society4 months agoAxiom-4 will see an Indian astronaut depart for outer space after 41 years
-

 Earth4 months ago
Earth4 months agoWorld Environment Day 2025: “Beating plastic pollution”
-

 Society6 months ago
Society6 months agoRabies, Bites, and Policy Gaps: One Woman’s Humane Fight for Kerala’s Stray Dogs